Javascript required
Skip to content Skip to sidebar Skip to footer
Home / Find All Solutions of Sinx Secx

Find All Solutions of Sinx Secx

Ways of solving trigonometric equations with detailed solutions are presented along with graphical interpretation.

Free Practice for SAT, ACT
and Compass Math tests

  1. Question

    Find all solutions of the equation
    sin(x) = 1 / 2
    in the interval [0 , 2π) and explain the solutions graphically using a unit circle and the graph of sin (x) - 1/2 in a rectangular coordinate system of axes.

    Solution


    sin(x) is positive in quadrant I and II and therefore the given equation has two solutions.
    In quadrant I, the solution to sin(x) = 1 / 2 is x = π / 6. (green in unit circle)
    In quadrant II, and by symmetry of the unit circle, the solution to sin(x) = 1 / 2 is x = π - π / 6 = 5π/6 (brown in unit circle)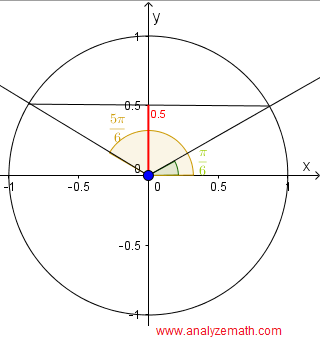

    sat question - graphical solution unit circle question 1 Pin it! Share on Facebook

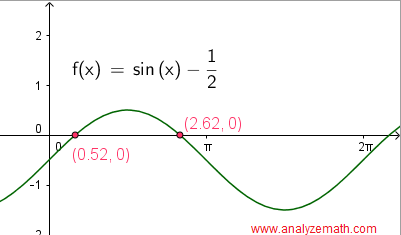
    The graphical solutions of the equation sin(x) = 1 / 2 are obtained by first writing the equation with right side equal to zero sin(x) - 1 / 2 = 0. Then graph the left side of the equation and the solutions of the equation are the x - intercepts of the graph of f(x) = sin(x) - 1 / 2 as shown below. It is easy to check that the two x intercepts in the graph below are close to the the analytical solutions found above:π / 6 and 5π/6.

    sat question - graphical solution question 1 Pin it! Share on Facebook

  2. Question

    Find all solutions of the equation
    sin(x) + cos(x) = 0
    in the interval [0 , 2π) and explain the solutions graphically using the unit circle and the graph of sin (x) + cos(x) in a rectangular coordinate system.

    Solution


    The equation may be written as: sin(x) = -cos(x).
    Divide both sides of the equation by cos(x): sin(x)/ cos(x) = -1
    Use the identity tan(x) = sin(x)/cos(x) to rewrite the equation as: tan(x) = - 1
    Solve the equation tan(x) = - 1
    tan(x) is negative in quadrant II and IV
    Solution in quadrant II: x = 3π/4
    By symmetry of the unit circle, the solution in quadrant IV is given by: 3π/4 + π = 7π / 4

    sat question - graphical solution unit circle question 2 Pin it! Share on Facebook

    The graphical solutions may be approximated by the x intercepts of the graph of g(x) = sin(x) + cos(x). We can easily check that the two solutions found above 3π/4 and 7π/4 are close to the x intercepts of the graph shown below.

    sat question - graphical solution question 2 Pin it! Share on Facebook

  3. Question

    Find all solutions of the equation:
    sin(πx / 2)cos(π/3) - cos(πx / 2)sin(π/3) = 0

    and check graphically the first few positive solutions.

    Solution


    We first use the formula sin(A - B) = sin A cos B - cos A sin B
    to write : sin(πx / 2)cos(π/3) - cos(πx / 2)sin(π/3) = sin(πx / 2 - π/3)
    rewrite the given equation as follows: sin(πx / 2 - π/3) = 0
    Solve the above equation to obtain: πx / 2 - π/3 = k π   where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...
    Simplify and rewrite the solution as: x = 2 k + 2/3   where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...

    sat question - graphical solution question 3 Pin it! Share on Facebook

    The first 3 positive solutions are: 2/3 , 8/3 and 14/3 corresponding to k =0, 1 and 2. These values are close to the x intercepts of the graph of f(x) = sin(πx / 2)cos(π/3) - cos(πx / 2)sin(π/3) shown above.

  4. Question

    Find all solutions of the equations: 2 sin2(x) + cos (x) = 1.
    and check graphically the first few positive solutions.

    Solution


    We first use the identity: sin2(x) = 1 - cos2(x)
    to rewrite the equation as: 2(1 - cos2(x)) + cos (x) = 1
    group like terms and write equation with right side equal to zero: -2 cos2(x) + cos (x) +1 = 0
    Let u = cos (x) and rewrite the equation as: -2 u2 + u +1 = 0
    Solve for u: u = 1 and u = - 1 / 2
    First group of solutions: Solve u = 1 for x: u = cos (x) = 1 :         x1 = 2 k π   where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...
    Solve u = -1 / 2 for x:
    cos(x) is negative in quadrant II and III, hence u = cos (x) = - 1/2 has two more groups of solutions given by
    Second group of solutions: x2 = 2π/3 + 2 k π   where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...
    Third group of solutions: x3 = 4π/3 + 2 k π   where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...

    sat question - graphical solution question 4 Pin it! Share on Facebook

    The first 3 positive solutions are obtained by setting k = 0 in all three groups of solutions to obtain: 0, 2π/3 and 4π/3. These values are close to the x intercepts of the graph of g(x) = 2 sin2(x) + cos (x) - 1 shown above.

  5. Question

    Find all solutions of the equation: 6 cos2(x / 2) - cos (x) = 4.
    and check graphically the first few positive solutions.

    Solution


    We first use the identity: cos(x) = 2 cos2(x / 2) - 1
    to rewrite the equation as: 6 cos2(x / 2) - (2 cos2(x / 2) - 1 ) = 4
    group like terms and write equation with right side equal to zero: 4 cos2(x / 2) = 3
    cos(x / 2) = ~+mn~ √3 / 2

    Solve the first equation cos(x / 2) = √3 / 2
    the cosine function is positive in quadrant I and IV hence the two groups of solutions:

    x / 2 = π / 6 + 2 k π where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ... (quadrant I)
    and
    x / 2 = 11π / 6 + 2 k π where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ... (quadrant VI)

    Multiply all terms by 2 to obtain the first two groups of solutions:

    x1 = π / 3 + 4 k π where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...
    x2 = 11π / 3 + 4 k π where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...

    Solve the second equation cos(x / 2) = - √3 / 2
    The cosine function is negative in quadrant II and III hence the two groups of solutions:

    x / 2 = 5 π / 6 + 2 k π where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ... (quadrant II)
    and
    x / 2 = 7 π / 6 + 2 k π where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ... (quadrant III)

    Multiply all terms by 2 to obtain two more groups two of solutions:

    x3 = 5 π / 3 + 4 k π where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...
    x4 = 7 π / 3 + 4 k π where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...

    sat question - graphical solution question 5 Pin it! Share on Facebook

    The first 4 positive solutions are obtained by setting k = 0 in all four groups of solutions to obtain: π/3 , 5π/3, 7π/3 and 11π/3. These values are close to the x intercepts of the graph of f(x) = 6 cos2(x / 2) - cos (x) - 4 shown above.

  6. Question

    Solve the equation: tan2 ( x) + 2 sec (x) + 1 = 0.
    and check graphically the first few positive solutions.

    Solution


    We first use the identity: tan2(x) = sec2(x) - 1
    to rewrite the equation as: sec2(x) - 1 + 2 sec (x) + 1 = 0
    group like terms and write equation with right side equal to zero: sec2(x) + 2 sec (x) = 0
    Factor: sec (x) (sec (x) + 2) = 0
    sec (x) = 0 has no solution.
    Solve : sec (x) + 2 = 0
    Use sec (x) = 1 / cos (x) to rewrite equation as : cos(x) = - 1 / 2

    The cosine function is negative in quadrant II and III hence two groups of solutions
    x1 = 2π/3 + 2 k π   where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...
    x2 = 4π/3 + 2 k π;   where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ...

    sat question - graphical solution question 6 Pin it! Share on Facebook


    The first 2 positive solutions are obtained by setting k = 0 in the two groups of solutions to obtain: 2π/3 and 4π/3. These values are close to the x intercepts of the graph of f(x) = tan2 ( x) + 2 sec (x) + 1 shown above.

  7. Question

    Solve the equation: cos(3x) + sin(2x) = cos(x).

    Solution


    We first use the identities:
    cos(3x) = cos3(x) - 3 cos(x)sin2(x)
    and
    sin(2x) = 2sin(x) cos(x)
    to rewrite the given equation as follows:      cos3(x) - 3 cos(x)sin2(x) + 2sin(x) cos(x) = cos(x)

    Rewrite with right hand side equal to zero and factor cos(x)
    cos(x) ( cos2(x) - 3 sin2(x) + 2 sin(x) - 1) = 0

    Use the identity cos2(x) = 1 - sin2(x) to rewrite equation as follows
    cos(x) (1 - sin2(x) - 3 sin2(x) + 2 sin(x) - 1) = 0

    Simplify
    cos(x) (- 4 sin2(x) + 2 sin(x) ) = 0

    Factor
    2 sin(x) cos(x) (1 - 2 sin(x)) = 0

    Set each factor equal to zero and solve.
    sin(x) = 0 gives solutions:           x1 = kπ
    cos(x) = 0 gives solutions:            x2 = π/2 + kπ
    1 - 2 sin(x) = 0 or sin(x) = 1/2 gives 2 other gropus of solutions.
    x3 = π/6 + 2kπ
    and
    x4 = 5π/6 + 2kπ

    sat question - graphical solution question 7 Pin it! Share on Facebook

    The first 6 positive solutions are obtained by setting k = 0 and k = 1 in the first two groups of solutions x1 and x2 to obtain: 0 , π, π/2, 3π/2, and k = 0 in the second and third groups of solutions x3 and x4 to obtain π/6 and 5π/6. These 6 solutions are close to the x intercepts of the graph of f(x) = cos(3x) + sin(2x) - cos(x) shown above.

  8. Question

    Solve the equation: cos(3x) = cos(2x + π/4).

    Solution


    We use the fact that if cos(A) = cos(B), then A = B + 2kπ or A = - B + 2kπ to write:

    3 x = 2 x + π/4 + 2kπ
    or
    3 x = -(2 x + π/4) + 2kπ

    Solve 3 x = 2 x + π/4 + 2kπ   Solutions:   x1 = π/4 + 2kπ
    Solve 3 x = -(2 x + π/4) + 2kπ ,
    gives 5x = - π/4 + 2kπ
    divide all terms by 5 : x2 = - π/20 + 2kπ / 5
    where   k = 0 , ~+mn~ 1 , ~+mn~ 2, ... in both groups of solutions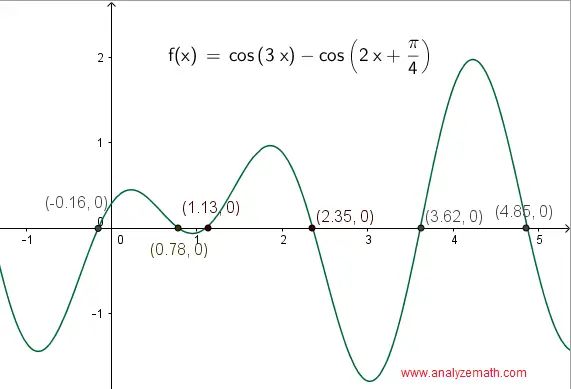

    sat question - graphical solution question 8 Pin it! Share on Facebook

    The 6 x intercepts shown in the graph of f(x) = cos(3x) - cos(2x + π/4) below correspond the solutions k = 0 in the first group x1 and k = 0, 1, 2 ,3 and 4 in the second group of solutions x2 = - π/20 + 2kπ / 5.

Find All Solutions of Sinx Secx

Source: https://www.analyzemath.com/high_school_math/grade_12/trigonometry_equations.html